RS Aggarwal Class 12 Solutions Chapter 9
RS Aggarwal Solutions for Class 12 Chapter 9 – Continuity and Differentiability Solutions PDF Download
RS Aggarwal Solutions Class 12 Maths Chapterwise – Free PDF Download
The RS Aggarwal Class 12 Solutions Chapter 9 Continuity and Differentiability are ready to help you answer all the questions. This chapter is the most important one about calculus. In the RS Aggarwal Solutions for Class 12 Chapter 9 – Continuity and Differentiability, you will learn what a continuous function is and how the domain of a function affects its continuity. You will also learn that the opposite of a continuous function and its modulus are also continuous functions too.
This Continuity and Differentiability in Class 12 RS Aggarwal is an important topic for students studying for the Class 12th Board exam. The textbook gives step-by-step answers to problems so that students can easily solve them. It will be a great way for them to practise and review, which will help them keep up with the growing competition. The ideas in this RS Aggarwal Class 12 Solutions Chapter 9 seem to be useful for future studies as well. The exercise is easy to use because it has questions and answers that are clear and easy to understand.
Click her to Buy Online RS Aggarwal Class 12 Book
RS Aggawal Class 12 Solutions Chapter 9 Continuity and Differentiability PDF





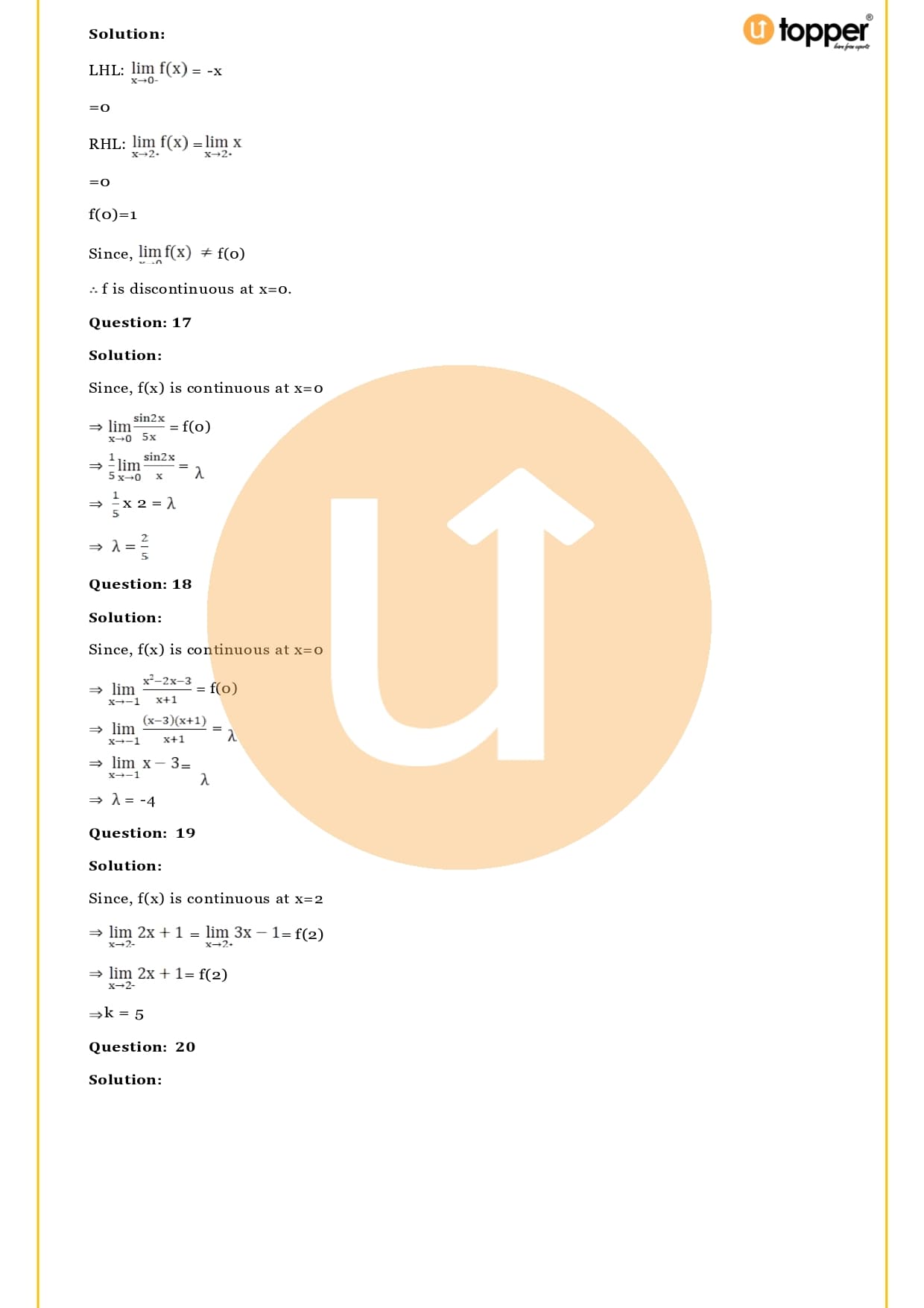
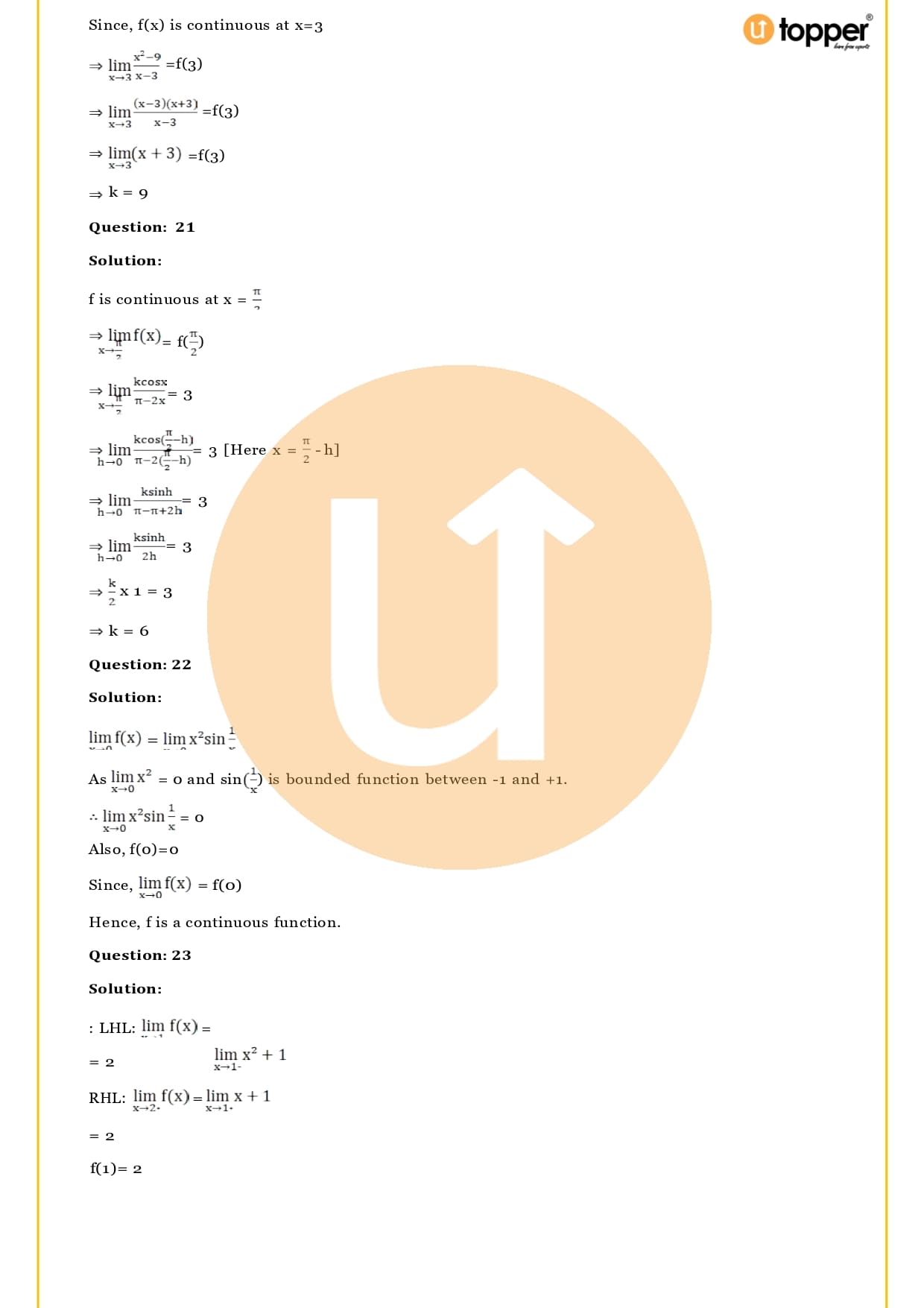

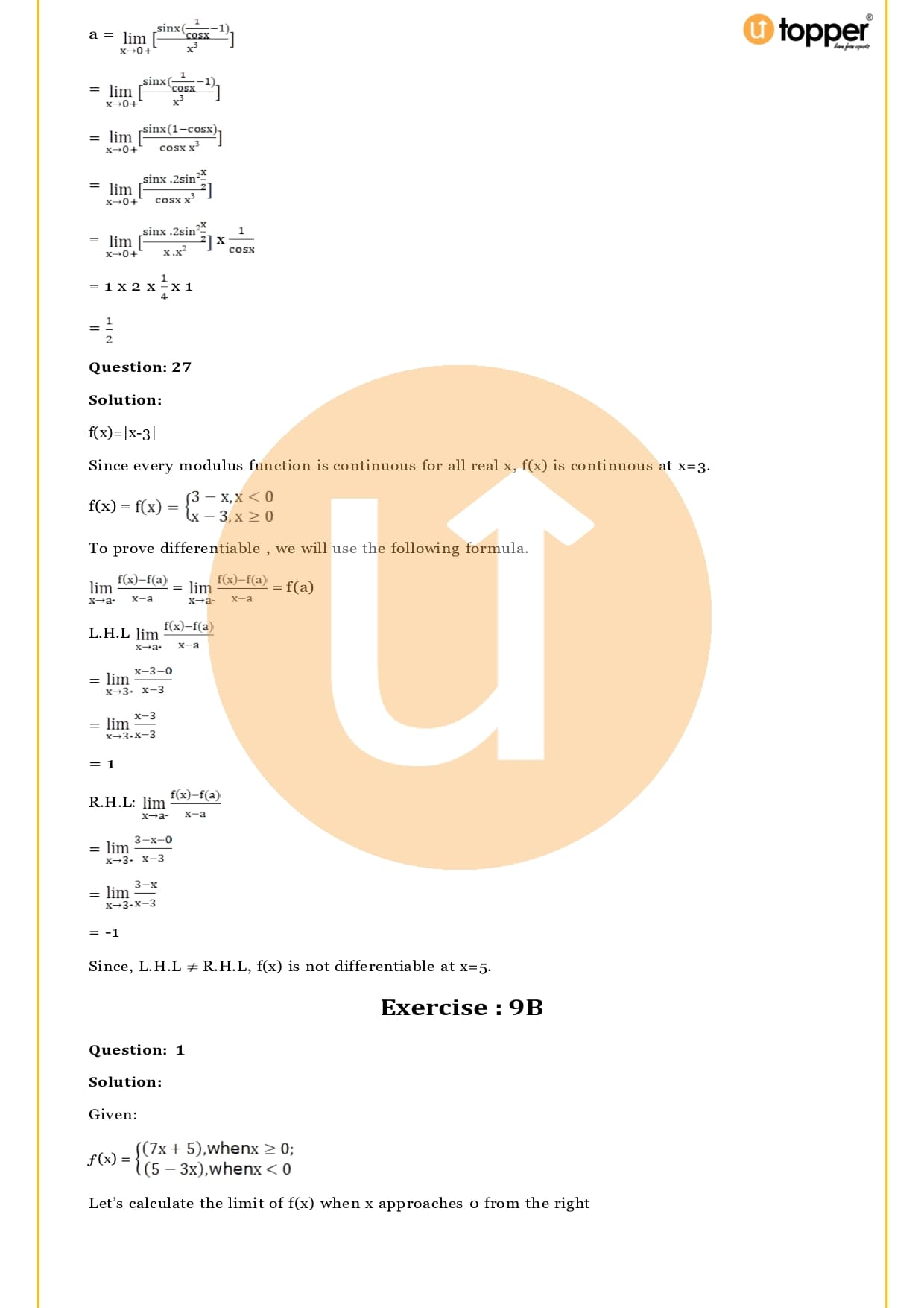












Summary of RS Aggarwal Solutions for Class 12 Chapter 9 “Continuity and Differentiability”
- In this chapter, we’ve talked about what it means for a function to be continuous if its graph doesn’t have any breaks or gaps between different points on a given domain.
- Graphs show the difference between a continuous function and a non-continuous function. Graphs are very important when learning about any function.
- It is possible to gain a better understanding of a wide range of mathematical concepts by practising the solutions that have been provided in advance.
- We will also study and prove the corollaries that follow from these theorems.
RS Aggarwal Class 12 Solutions
Chapter-1 Relation Solutions
Chapter-2 Functions Solutions
Chapter-3 Binary Operations Solutions
Chapter-4 Inverse Trigonometric Functions Solutions
Chapter-5 Matrices Solutions
Chapter-6 Determinants Solutions
Chapter-7 Adjoint and Inverse of a Matrix Solutions
Chapter-8 System of Linear Equations Solutions
Chapter-9 Continuity and Differentiability Solutions
Chapter-10 Differentiation Solutions
Chapter-11 Applications of Derivatives Solutions
Chapter-12 Indefinite Integral Solutions
Chapter-13 Method of Integration Solutions
Chapter-14 Some Special Integrals Solutions
Chapter-15 Integration Using Partial Fractions Solutions
Chapter-16 Definite Integrals Solutions
Chapter-17 Area of Bounded Regions Solutions
Chapter-18 Differential Equations and Their Formation Solutions
Chapter-19 Differential Equations with Variable Separable Solutions
Chapter-20 Homogeneous Differential Equations Solutions
Chapter-21 Linear Differential Equations Solutions
Chapter-22 Vectors and Their Properties Solutions
Chapter-23 Scalar, or Dot, Product of Vectors Solutions
Chapter-24 Cross, or Vector, Product of Vectors Solutions
Chapter-25 Product of Three Vectors Solutions
Chapter-26 Fundamental Concepts of 3-Dimensional Geometry Solutions
Chapter-27 Straight Line in Space Solutions
Chapter-28 The Plane Solutions
Chapter-29 Probability Solutions
Chapter-30 Bayes’s Theorem and its Applications Solutions
Chapter-31 Probability Distribution Solutions
Chapter-32 Binomial Distribution Solutions
Chapter-33 Linear Programming Solutions

RS Aggarwal Class 12 Solutions Chapter 9 Continuity and Differentiability in PDF Format
In Class 12 Chapter 9 of Aggarwal, which is all about the idea of Continuity and Differentiability, there are a total of 3 exercises. In Exercise 9A, there are questions that ask students to prove the conditions that show if the function is continuous or not. In Exercise 9B, students see more complex functions that are harder to understand. In Exercise 9C, there are questions that ask students to check whether the functions stay the same or change. The questions cover things like how to prove different theorems and how to tell the difference between continuous and discontinuous functions by looking at graphs.
Concept of Continuity and Differentiation
- Continuity and Differentiation, which is explained in RS Aggarwal Class 12 Solutions Chapter 12, is one of the most important ideas for students studying for board exams.
- It talks about things like the derivative of functions, the continuity of certain points, the continuity of given intervals, and more.
- The values and characteristics of a function are based on how long it stays the same.
- In a certain range or domain, a function is considered to be continuous if the curve doesn’t break or have any missing points. In other words, the curve must be the same at every point in its domain.
Here are some of the most important things about RS Aggarwal Class 12 Solution Chapter 9:
- The answers to all the questions in Chapter 9 of Class 12 Maths are made by our subject-matter experts, who have years of experience in this field.
- These answers are prepared by the experts of Utopper who have more years of teaching experience.
- The answers are written in easy-to-understand language and step-by-step order, which will help students learn the basics of calculus.
- By doing these questions over and over, you’ll be able to get better grades and become a better thinker.
- Solutions of every chapter are well categorized to enhance the convenience of use during your revisions.
FAQ ( Frequently Asked Questions )
1. What are some key ideas to learn from RS Aggarwal Class 12 Solutions Chapter 9 Continuity and Differentiability?
Ans – In Class 12 RS Aggarwal chapter “Continuity and Differentiability,” there are questions about how to show that an equation is continuous when given different values of x.
Here are some of the most important parts of the chapter:
- Continuous functions have sums, differences, quotients, and products that are also continuous.
- At a given point, the differentiable function can be continuous, but the opposite may not always be true.
- Rolle’s Theorem says that a function f: x,y R is continuous on x and y and differentiable on x and y if f(x) = f(y) (y). There should also be a point z in the middle of (x,y), and f(z) should equal 0.
- Mean Value Theorem: If f: x,y R is continuous on x and y and differentiable on x and y. There should also be a point z between x and y, and f(z) should equal (f(y) – f(x))/. (y-x).
2. Where can I find revision notes for chapter 9 of CBSE class 12 math book Differential Equations?
Ans – Students can get the Class 12 Math revision notes for free on the Utopper website, where they can also find other useful information. Students can get free study materials from Utopper that they can download and use whenever they want on any device. Expert teachers put together these review notes. They cover all of the chapter’s ideas, as well as any important formulas and examples that will help students understand them better.
3. How Do RS Aggarwal Class 12 Solutions Chapter 9 Exercises Help Me Study for an Exam?
Ans – Students in CBSE who are studying for their 12th Boards will benefit from the RS Aggarwal Solutions for Class 12 Chapter 9.
- It will give a short analysis of the conditions that must be met to prove whether a function is continuous or discontinuous.
- In the Chapter 9 Solutions exercise, you can check the continuity of functions that are a bit more complicated.
- As you move through RS Aggarwal Class 12 solutions chapter 9, think about a function’s continuity and its ability to be differentiated.
- Also, the questions in the exercises are set up like the questions on competitive exams. This will help the students get ready ahead of time and improve their ability to think critically.
- Students studying for boards need to figure out how to answer these questions if they want to do better on the test.

