RS Aggarwal Class 11 Solutions Chapter 11
RS Aggarwal Solutions for Class 11 Chapter 11 – Arithmetic Progression PDF
RS Aggarwal Class 11 Solutions Chapter-wise – Free PDF Download
The best study guide for you is RS Aggarwal Class 11 Solutions Chapter 11: Arithmetic Progression. It will help you understand how to solve arithmetic progression questions on exams. This chapter covers some important ideas, like sequence, series, the properties of an arithmetic progression, the sum of n terms of an arithmetic progression, and the arithmetic mean. There are 6 exercises and about 100 practise questions in this chapter.
A student might not find it easy to answer all 100 questions in the chapter. So, Utopper experts made RS Aggarwal Chapter 11 Solutions for them. These answers will help you figure out everything you don’t understand about the chapter. They will also help you when it’s time to study again. Just look for a question you find hard, figure out how to solve it right, and do it as many times as you want. All of the answers are based on the CBSE exam pattern, so you can easily use them to prepare for your exam.
Click here to Buy Online The RS Aggarwal Class 10 Book Now
RS Aggarwal Class 11 Solutions Chapter 11 – Arithmetic Progression
















































Topics that are important for RS Aggarwal Class 11 Solutions Chapter 11
A sequence is a function whose domain is a subset of the natural numbers. A sequence is called a real sequence if its range is a subset of R (real numbers). If the sum of a sequence’s first n terms looks like An2 + Bn, where A and B are constants, then the sequence is called an arithmetic progression, or AP. The difference between this AP and the rest is 2A.
- If you add or take away a constant from each term of an AP, you get another AP with the same common difference.
- If we multiply or divide each term of an AP by a non-zero constant, the resulting sequence will also be an AP.
- If terms a, b, and c are all in the same AP, then 2b = a+c.
RS Aggarwal Class 11 Solutions
Chapter 1: Sets
Chapter 2: Relations
Chapter 3: Functions
Chapter 4: Principle of Mathematical Induction
Chapter 5: Complex Numbers and Quadratic Equations
Chapter 6: Linear Equations in One Variable
Chapter 7: Linear Equations in Two Variable
Chapter 8: Permutations
Chapter 9: Combinations
Chapter 10: Binomial Theorem
Chapter 11: Arithmetic Progression
Chapter 12: Geometrical Progression
Chapter 13: Some Special Series
Chapter 14: Measurement of Angles
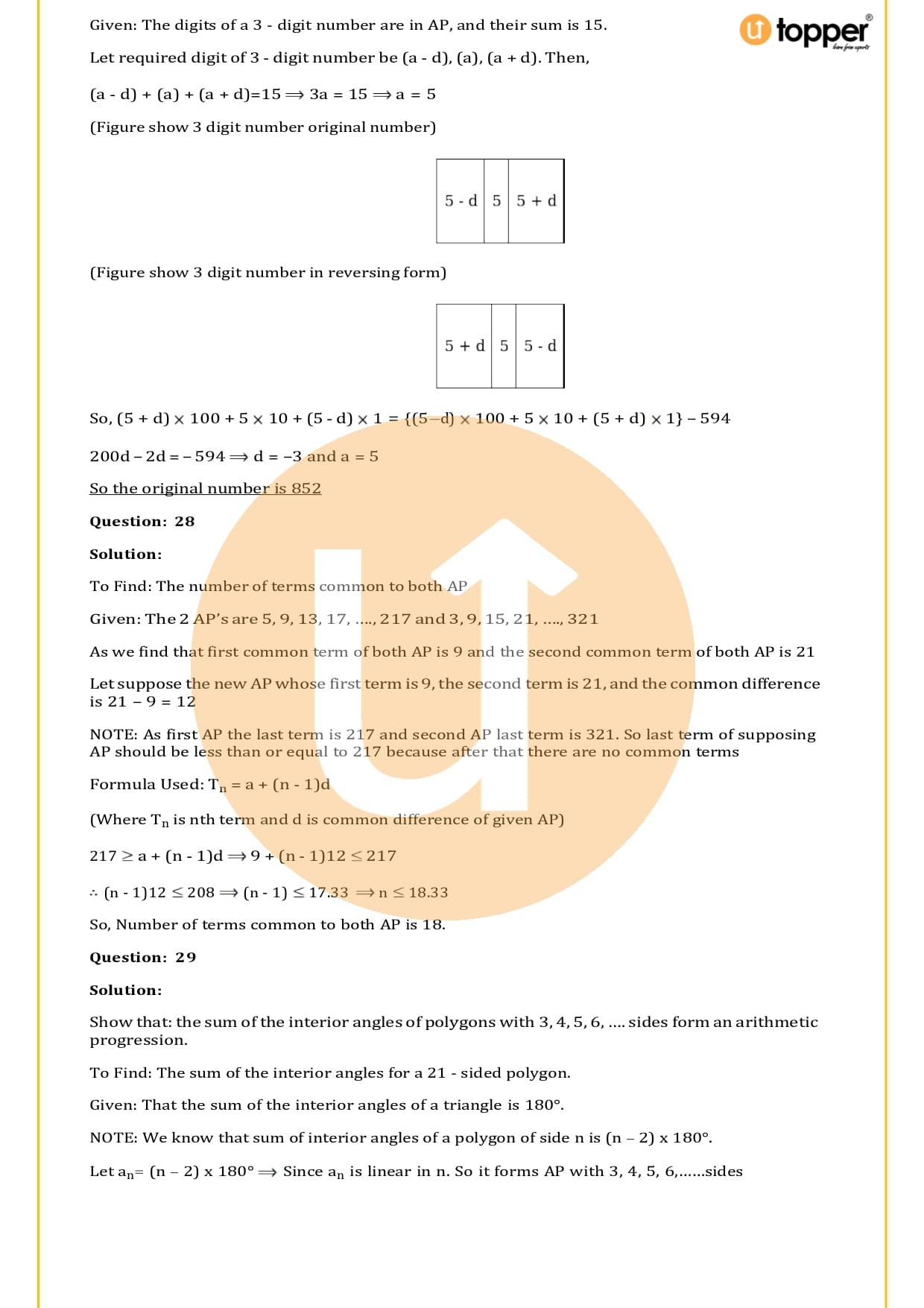
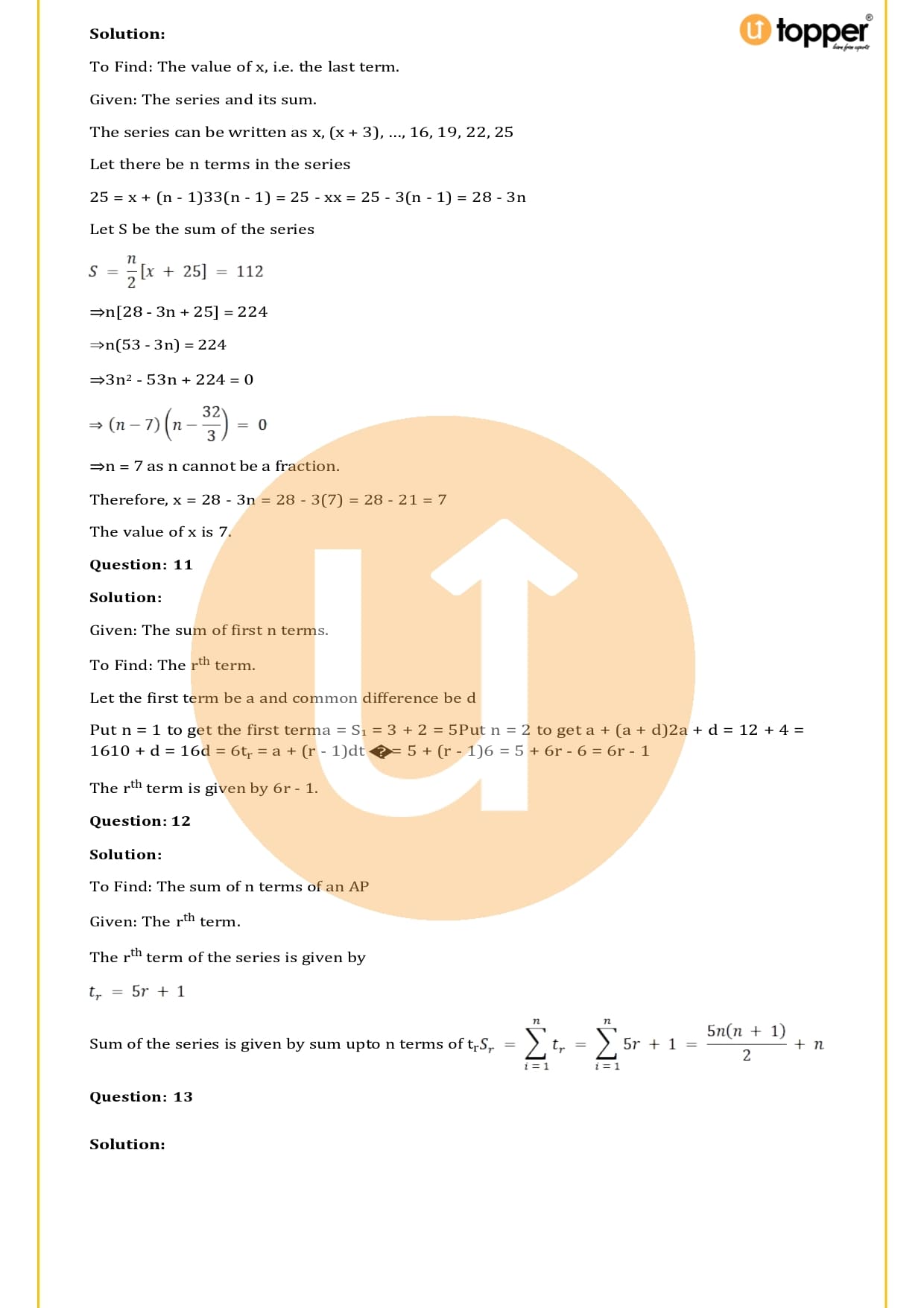
Chapter 15: Trigonometric or Circular Functions
Chapter 16: Conditional Identities Involving the Angles of a Triangles
Chapter 17: Trigonometric Equations
Chapter 18: Solution of Triangles
Chapter 19: Graphs of Trigonometric Functions
Chapter 20: Straight Lines
Chapter 21: Circles
Chapter 22: Parabola
Chapter 23: Ellipse
Chapter 24: Hyperbola
Chapter 25: Applications of Conic Sections
Chapter 26: Three Dimensional Geometry
Chapter 27: Limits
Chapter 28: Differentiation
Chapter 29: Mathematical Reasoning
Chapter 30: Statistics
Chapter 31: Probability

Arithmetic Progression RS Aggarwal Class 11 Solutions Chapter 11
A progression is a series of events that happen in a certain order and follow certain rules. Arithmetic Progression is an important part of RS Aggarwal Class 11 Solutions Chapter 11. It is a series of numbers in which each number adds a constant amount to the number before it. It says that the difference between the term before and the term after is always the same amount. For example, the difference between any two natural numbers (1, 2, 3, 4, 5, 6, etc.) is always 1. Also, the difference between odd and even numbers always stays at 2.
Why is learning arithmetic progression helpful?
An arithmetic progression is a list of terms that have a constant difference between them. It’s a word that’s used to describe a group of patterns we see every day.
A key mathematical skill is being able to recognise and create patterns, which helps us make predictions based on what we see. Pattern recognition helps children learn and understand more complicated mathematical ideas and procedures. Patterns help us see connections and draw broad conclusions.
What are the properties of RS Aggarwal Class 11 Solutions Arithmetic Progression?
In RS Aggarwal class 11 Solutions chapter 11 , these are the properties of arithmetic progression that are talked about:
- Property 1: If you add or subtract any constant term from the given AP in each number, the resulting sequence will be an arithmetic progression.
- Property 2: When each term of a given AP is multiplied or divided by a constant that is not zero, the result is also an arithmetic progression.
- Property 3: In an arithmetic progression with a finite number of terms, the sum of two numbers that are the same distance from the end and the beginning is the same as the sum of the last term and the first term.
- Property 4: The three numbers are in an arithmetic progression if 2b = a+c.
- Property 5: If the nth term of a sequence is a linear expression, then the sequence is an arithmetic progression.
- Property 6: A sequence is an arithmetic progression if the sum of the first n terms is An2 + Bn, where A and B are constants that don’t change with n.
- When a constant is added or subtracted from each term of an AR, the result is an AP with the same common difference. If each term of an AP is multiplied or divided by a number that is not zero, the result is also an AP.

FAQ ( Frequently Asked Questions )
1. How to Get Good Marks in Arithmetic Progression?
Ans – Students need to spend a lot of time practising arithmetic progression in order to study for tests and get good grades. It will help them figure out how to solve problems and get rid of any mistakes in facts. Always keep the different ideas and formulas of arithmetic progression close at hand so you can quickly figure out the answer. This will help you get through the test faster. For this, students have to do different exercises and figure out how to answer all the questions.
This will get them used to the different amounts in the exercises. First, you need to understand the concept. Then, you need to practise to get ready for the test. And other aspects for getting good marks you can access free study materials like Revision Notes, Important questions and many more.
2. What are some other kinds of mathematical progressions?
Ans – Harmonic Progression and Geometric Progression are two other types of progression. Progression of Harmony.
When multiplying the reciprocals of an arithmetic progression, a harmonic progression (or harmonic sequence) is formed. A sequence is the same as a harmonic progression if each term is the harmonic mean of the terms around it. Geometric Progression, on the other hand, is a series in which each new element is made by multiplying the previous element by a constant called the common ratio, which is represented by the letter r.
3. What does the term Series in Arithmetic Progression mean?
Ans – A mathematical series is the sum of a arithmetic sequence. We find the sum by adding the first and last terms, a1 and an, dividing by 2 to get the average of the two numbers, and then multiplying by the number of numbers, n: Sn = n2(a1+an). Any part of our lives can be thought of in terms of arithmetic progression or sequence. Now all we have to do is look at how we use it every day. When we know about this kind of sequence, we can see how things happen in our lives from a different point of view.
