Types of Decimal Representations:
Any rational number can have two types of decimal representations (expansions):
- Terminating
- Non-terminating but repeating
Any irrational number can have only one type of decimal representation (expansion):
- Non-terminating and Non-repeating
The exactness of decimal representations of Irrational Numbers:
Let’s have a look at the decimal representation of the following irrational numbers:
√2=1.4142135623…
π=3.1415926535…
Clearly, these are the non-terminating and non-repeating decimal expansion that has an infinite number of digits and there is no pattern as well.
What does this really mean? Does this mean that we do not know the exact value of irrational numbers as we do for rational numbers? No, that’s not correct! When we talk about an irrational number, say,
√2 or π, we do know the exact number (or quantity) we are talking about. For example, we can exactly construct a length of
√2 units, given a length of 1 unit. We know that the ratio of the circumference to the diameter in any circle is exactly equal to π.
Well, you should think of this as follows: the decimal representation of a number is just one way to represent a number. In the case of irrational numbers, it so happens that their decimal representations are non-terminating and non-repeating. But this does not mean that irrational numbers are not exact in any sense. It only means that when we try to write an irrational number in decimal form, the sequence of digits after the decimal point never ends.
How to represent irrational numbers exactly?
Let’s think of \(\sqrt 2 \) for a moment. If we try to write \(\sqrt 2 \) in decimal form (say, to 5 decimal digits), we have \(\sqrt 2 = 1.41421 \ldots \). Another person might say: Let me specify \(\sqrt 2 \) more accurately, say up to 10 decimal digits. He would write
\[\sqrt 2 = 1.4142135623\ldots \]
Still another person might decide to write \(\sqrt 2 \) even more accurately, to a thousand decimal digits, for example. The more digits we include in the representation, the closer our number is to the actual value of \(\sqrt 2 \). But what is the actual value? Do we even know it? Can we represent it somehow (by doing something other than just writing \(\sqrt 2 \))?
Yes, we can – geometrically!
If we construct a right-angled triangle with the two sides each of length 1 unit, the hypotenuse is exactly \(\sqrt 2 \) units:
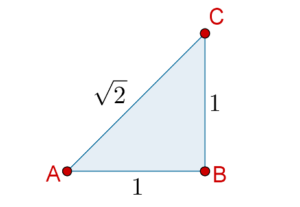
The geometrical representation is correct, however, the numeric representation may be imprecise (regardless of the number of digits used).
To sum up the entire discussion:
If no significant digits are omitted, the decimal representation of a rational number is precise. This is due to the fact that it will be either terminating or non-terminating but repeated.
The decimal representation of an irrational number will always be imprecise because it will never end and will never recur.
Solved Examples :
Example 1: What is \(\pi \)?
Solution: \(\pi \) is an irrational number and the ratio of the circumference to the diameter in any circle.
The approximate value of \(\pi \) is \(\pi \approx \frac{{22}}{7}\). This is approximate, not exact. Since \(\pi \) is not a rational number, it cannot be exactly specified in \(\frac{p}{q}\) form. In decimal form, we can write approximately as \(\pi \approx 3.14159\).
The value \(\frac{22}{7}\) is only a rational approximation for \(\pi \), which is actually an irrational number. In fact, a better rational approximation of \(\pi \) is \(\frac{355}{113}\).

